Navigation:
UCSB Seal

Paul J. Atzberger

  • Department
    • Applied Mathematics
    • Employment/Positions
    • Event Calendar
    • People
      • Staff
      • Faculty
      • Visitors
    • Directions
    • UCSB Map
  • Research
    • Course Notes and Resources
    • Math Research Groups
      • Applied Math
      • Analysis
      • Partial Differential Equations
      • Geometry
      • Algebra
      • Topology
      • Number Theory
    • UCSB Research Groups
      • Kavli Institute (KITP Physics)
      • Materials Research (MRL)
      • California Nanosystems Institute (CNSI)
      • Computer Science and Engineering (CSE)
      • Center for Financial Mathematics and Statistics (CRFMS)
    • UCSB Math Preprint Server
    • Science Direct
    • Math Sci Net
    • LALN arXiv
    • CiteSeer IST
    • ISI Web of Knowledge
  • Graduate
    • Prospective Students
  • Undergraduate
    • Prospective Students

Homepage Curriculum Vitae Δ Publications Research Summary Software Teaching Intranet Applied Mathematics Group Positions Available

Attach:photo_bk.jpg Δ

Fluctuating Hydrodynamics and Fluid-Structure Interactions Subject to Thermal Fluctuations

Thermal fluctuations and entropic effects play a significant role at the small physical length, time, and energy scales often relevant to phenomena in soft materials, biological systems, and microfabricated devices. Hydrodynamic theories often offer an effective kinetic description of the important roles played by solvent mediated momentum transfer that couples the motions of microstructures. To incorporate the important role of fluctuations we have developed new fluctuating hydrodynamic approaches both for theoretical investigations and computational simulations. This includes the Stochastic Immersed Boundary Method (SIB) for the efficient numerical study of dynamics of elastic structures which interact with a hydrodynamic flow subject to thermal fluctuations. We have also introduced a general framework for development of such methods referred to as the Stochastic Eulerian Lagrangian Method (SELM). We are also working on specific scientific applications with these approaches (see below research for more details).

Computational Fluid Dynamics : Fluid-Structure Interaction : Stochastic Immersed Boundary Methods

The Stochastic Immersed Boundary Method (SIB) is a numerical approach for studying the mechanics of elastic structures which interact with a fluid in the presence of thermal fluctuations. The hydrodynamic interactions of the composite system are handled by an approximate treatment of the fluid-structure stresses. This approximation is expressed in terms of a Lagrangian representation of the immersed structures which is coupled to an Eulerian representation of the fluid. Thermal fluctuations are accounted for in the system by an appropriate stochastic forcing of the fluid-structure equations in accordance with the principles of statistical mechanics. The formalism is cast in terms of a system of Stochastic Partial Differential Equations (SPDE's). Fast time scales introduced into the fluid dynamics by the thermal fluctuations pose a challenge for conventional approaches to numerical approximation. Using results from stochastic calculus, we have developed efficient stochastic numerical methods for this formalism. We are currently extending this formalism to include more general coupling operators for approximation of the fluid-structure interactions with thermal fluctuations. This includes operators which allow for more general representations of immersed structures and which account for translational and rotational motions of immersed structures. We are also developing new stochastic numerical methods for non-periodic domains and adaptive multilevel meshes. For more details, see the papers and preprints below.

⚠ (:area shape=rect coords=300,0,398,102 href=http://www.math.ucsb.edu/~atzberg/movies/unknot.avi:) ⚠ (:area shape=rect coords=398,0,499,102 href=http://www.math.ucsb.edu/~atzberg/movies/trefoilKnot.avi :) ⚠ (:area shape=rect coords=499,0,600,102 href=http://www.math.ucsb.edu/~atzberg/movies/figureEightKnot.avi :) ⚠ (:area shape=rect coords=300,103,398,187 href=http://www.math.ucsb.edu/~atzberg/movies/membraneSheet.avi" :) ⚠ (:area shape=rect coords=398,103,499,187 href=http://www.math.ucsb.edu/~atzberg/movies/membraneVesicle.avi" :) ⚠ (:area shape=rect coords=499,103,600,187 href=http://www.math.ucsb.edu/~atzberg/movies/membraneVesicleFormation.avi" :) ⚠ (:area shape=rect coords=414,205,473,253 href=http://www.math.ucsb.edu/~atzberg/movies/motor_no_load.avi" :) ⚠ (:area shape=rect coords=473,205,529,253 href=http://www.math.ucsb.edu/~atzberg/movies/motor_moderate_load.avi" :) ⚠ (:area shape=rect coords=529,205,600,253 href=http://www.math.ucsb.edu/~atzberg/movies/motor_large_load.avi" :)

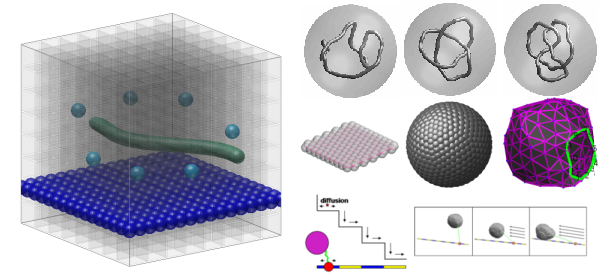
Immersed structures in SIB can be used to represent the mechanics of a variety of microscopic hydrodynamic systems. We have applied the approach to a number of applications, see the figure above and movies below. In the context of complex fluids, immersed structures have been used to represent solute particles, polymers, or membrane structures. We have also extended the SIB approach to study the static and dynamic rheological responses of a number of complex fluids, see the papers below. We are also developing dynamic coarse-grained models of lipid bilayer membranes using the SIB formalism to account for molecular level interactions, lipid-lipid and lipid-solvent hydrodynamic coupling, and thermal fluctuations, see the papers and talks below.

Select Publications

  • Stochastic Eulerian-Lagrangian Methods for Fluid-Structure Interactions with Thermal Fluctuations, Atzberger, P.J., (2010), (preprint). [PDF]
  • Simulation of Complex Fluids using Stochastic Eulerian Lagrangian Methods with Shear Boundary Conditions, Atzberger, P.J., (2010), (preprint). [PDF]
  • Spatially Adaptive Stochastic Multigrid Methods for Fluid-Structure Systems with Thermal Fluctuations, Atzberger, P.J., (preprint), (2010). [PDF]
  • A Stochastic Immersed Boundary Method for Fluid-Structure Dynamics at Microscopic Length Scales, Atzberger, P.J., Kramer, P.R., and Peskin, C.S., J. Comp. Phys., Vol. 224, Iss. 2, (2007). [PDF] [DOI]
  • Stochastic Immersed Boundary Method Incorporating Thermal Fluctuations (brief introduction), Atzberger, P.J., Kramer, P.R., and Peskin, C.S., (proceedings of ICIAM 2007). [PDF] [DOI]
  • A Note on the Correspondence of the Immersed Boundary Method with Thermal Fluctuations To Stokesian-Brownian Dynamics, Atzberger, P.J., Physica D, Vol. 226, Iss. 2, 15, pg. 144-150, (2007). [PDF] [DOI]
  • On the Foundations of the Stochastic Immersed Boundary Method, Kramer, P.R., Peskin, C.S., and Atzberger, P.J., Comp. Meth. in Appl. Mech. and Eng., Vol. 197, Iss. 25-28, 15 April, pp. 2232-2249, (2008). [PDF] [DOI]
  • Dynamic Implicit-Solvent Coarse-Grained Models of Lipid Bilayer Membranes : Fluctuating Hydrodynamics Thermostat, Y. Wang, J. K. Sigurdsson, E. Brandt, and P.J. Atzberger (preprint), (2012). [PDF]

Movies for Select Simulation Results

  1. Simulations of polymer knots showing SIB preservation of knot topology without the need for excluded volume interactions
    • Unknotted Polymer [MPG]
    • Trefoil Knot [MPG]
    • Figure Eight Knot [MPG].
  2. Simulations demonstrating a tethered membrane model using SIB for the hydrodynamic coupling
    • Membrane Sheet [AVI]
    • Membrane Vesicle [AVI]
    • Membrane Vesicle Formation [AVI].
  3. Simulations of motor protein transporting a cargo vesicle under an imposed hydrodynamic load
    • No Load [AVI]
    • Intermediate Load [AVI]
    • Large Load [AVI].



⚠ <iframe title="YouTube video player" class="youtube-player" type="text/html" width="200" height="200" src="http://www.youtube.com/embed/EInd4Pptm_A?controls=0&hl=en&loop=1" controls="0" showinfo="0" frameborder="0" allowFullScreen> ⚠ </iframe> ⚠ <iframe title="YouTube video player" class="youtube-player" type="text/html" width="200" height="200" src="http://www.youtube.com/embed/r86K4Bkzlzs?hl=en&fs=1&controls=0&loop=1" frameborder="0" allowFullScreen> ⚠ </iframe> ⚠ <iframe title="YouTube video player" class="youtube-player" type="text/html" width="200" height="200" src="http://www.youtube.com/embed/j6d0-WBXUFM?hl=en&fs=1&controls=0&loop=1" frameborder="0" allowFullScreen> ⚠ </iframe>



⚠ <iframe title="YouTube video player" class="youtube-player" type="text/html" width="200" height="200" src="http://www.youtube.com/embed/-ipANLU7cCA?controls=0&hl=en&loop=1" controls="0" showinfo="0" frameborder="0" allowFullScreen> ⚠ </iframe> ⚠ <iframe title="YouTube video player" class="youtube-player" type="text/html" width="200" height="200" src="http://www.youtube.com/embed/_bDZx0AT8Bw?hl=en&fs=1&controls=0&loop=1" frameborder="0" allowFullScreen> ⚠ </iframe> ⚠ <iframe title="YouTube video player" class="youtube-player" type="text/html" width="200" height="200" src="http://www.youtube.com/embed/PyE2FX7eegs?hl=en&fs=1&controls=0&loop=1" frameborder="0" allowFullScreen> ⚠ </iframe>



⚠ <iframe title="YouTube video player" class="youtube-player" type="text/html" width="200" height="200" src="http://www.youtube.com/embed/jwIhZNA4-lI?hl=en&fs=1&controls=0&loop=1" frameborder="0" allowFullScreen> ⚠ </iframe> ⚠ <iframe title="YouTube video player" class="youtube-player" type="text/html" width="200" height="200" src="http://www.youtube.com/embed/9YDcSLB9nVM?hl=en&fs=1&controls=0&loop=1" frameborder="0" allowFullScreen> ⚠ </iframe> ⚠ <iframe title="YouTube video player" class="youtube-player" type="text/html" width="200" height="200" src="http://www.youtube.com/embed/gOkD2OofxbY?hl=en&fs=1&controls=0&loop=1" frameborder="0" allowFullScreen> ⚠ </iframe>



⚠ <iframe title="YouTube video player" class="youtube-player" type="text/html" width="200" height="200" src="http://www.youtube.com/embed/w61e1SElxzE?hl=en&fs=1&controls=0&loop=1" frameborder="0" allowFullScreen> ⚠ </iframe> ⚠ <iframe title="YouTube video player" class="youtube-player" type="text/html" width="200" height="200" src="http://www.youtube.com/embed/Ha8yIim4xJM?hl=en&fs=1&controls=0&loop=1" frameborder="0" allowFullScreen> ⚠ </iframe> ⚠ <iframe title="YouTube video player" class="youtube-player" type="text/html" width="200" height="200" src="http://www.youtube.com/embed/Y8LuFMALNqI?hl=en&fs=1&controls=0&loop=1" frameborder="0" allowFullScreen> ⚠ </iframe>



Edit | History | Print | Recent Changes | Edit Sidebar

Page last modified on August 02, 2013, at 07:32 pm


Contact Us